Second Order Kinetics Half Life
two.8: 2nd-Order Reactions
- Folio ID
- 1440
Many important biological reactions, such as the formation of double-stranded DNA from two complementary strands, tin be described using second guild kinetics. In a second-club reaction, the sum of the exponents in the charge per unit law is equal to two. The two most common forms of second-lodge reactions volition be discussed in particular in this section.
To draw how the charge per unit of a 2d-order reaction changes with concentration of reactants or products, the differential (derivative) rate equation is used also as the integrated rate equation. The differential rate police tin show the states how the rate of the reaction changes in time, while the integrated charge per unit equation shows how the concentration of species changes over time. The latter form, when graphed, yields a linear role and is, therefore, more than convenient to look at. Nonetheless, both of these equations can exist derived from the above expression for the reaction rate. Plotting these equations tin also assist us decide whether or not a certain reaction is second-gild.
Case 1: Identical Reactants
Two of the same reactant (\(\ce{A}\)) combine in a single elementary step.
\[\begin{align} \ce{A} + \ce{A} &\ce{->} \ce{P} \label{case1a} \\[4pt] \ce{2A} &\ce{->} \ce{P} \label{case1b} \terminate{align}\]
The reaction rate for this footstep can exist written as
\[\text{Rate} = - \dfrac{1}{ii} \dfrac{d[A]}{dt} = + \dfrac{d[P]}{dt} \nonumber\]
and the rate of loss of reactant \(\ce{A}\)
\[\dfrac{dA}{dt}= -k[A][A] = -k[A]^ii \label{2ndlaw}\]
where \(chiliad\) is a second club charge per unit constant with units of Chiliad-1 min-1 or M-1 south-1. Therefore, doubling the concentration of reactant \(\ce{A}\) volition quadruple the charge per unit of the reaction. In this item instance, another reactant (\(B\)) could be present with \(A\); however, its concentration does non touch on the rate of the reaction, i.east., the reaction lodge with respect to B is zero, and nosotros can express the charge per unit law every bit \(v = k[A]^two[B]^0\).
Integration of Equation \ref{2ndlaw} yields
\[ \dfrac{one}{[A]} = \dfrac{1}{[A]_0}+kt\]
which is easily rearranged into a form of the equation for a straight line and yields plots similar to the one shown beneath.
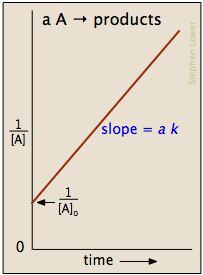
The half-life is given past
\[ t_{1/2}=\dfrac{1}{k[A_o]}\]
Notice that the half-life of a second-order reaction depends on the initial concentration, in contrast to commencement-order reactions. For this reason, the concept of half-life for a 2d-order reaction is far less useful. Reaction rates are discussed in more detail here. Reaction orders are defined here. Here are explanations of zero and commencement gild reactions.
For reactions that follow Equation \ref{case1a} or \ref{case1b}, the rate at which \(\ce{A}\) decreases tin be expressed using the differential rate equation.
\[-\dfrac{d[A]}{dt} = k[A]^2\]
The equation can then exist rearranged:
\[\dfrac{d[A]}{[A]^2} = -k\,dt\]
Since nosotros are interested in the change in concentration of A over a period of time, nosotros integrate between \(t = 0\) and \(t\), the time of interest.
\[ \int_{[A]_o}^{[A]_t} \dfrac{d[A]}{[A]^2} = -thou \int_0^t dt\]
To solve this, we use the following dominion of integration (power rule):
\[\int \dfrac{dx}{ten^2} = -\dfrac{i}{x} + constant\]
We and so obtain the integrated rate equation.
\[\dfrac{one}{[A]_t} - \dfrac{ane}{[A]_o} = kt\]
Upon rearrangement of the integrated rate equation, we obtain an equation of the line:
\[\dfrac{1}{[A]_t} = kt + \dfrac{1}{[A]_o}\]
The crucial part of this process is non understanding precisely how to derive the integrated rate police equation, rather it is important to understand how the equation directly relates to the graph which provides a linear relationship. In this case, and for all second order reactions, the linear plot of \(\dfrac{i}{[A]_t}\) versus time will yield the graph below.
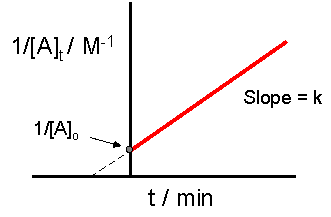
This graph is useful in a variety of ways. If we only know the concentrations at specific times for a reaction, nosotros tin attempt to create a graph like to the one above. If the graph yields a straight line, then the reaction in question must exist second order. In addition, with this graph we can notice the slope of the line and this slope is \(k\), the reaction constant. The slope tin be institute be finding the "rising" and and so dividing it by the "run" of the line. For an example of how to observe the slope, please see the example section below. There are culling graphs that could be drawn.
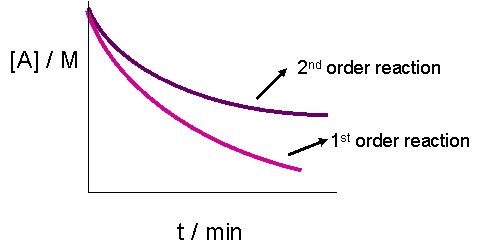
The plot of \([A]_t\) versus fourth dimension would upshot in a straight line if the reaction were zeroth order. It does, however, yield less information for a second social club graph. This is considering both the graphs of a first or 2nd order reaction would look similar exponential decays. The only obvious difference, every bit seen in the graph below, is that the concentration of reactants approaches aught more than slowly in a 2nd-order, compared to that in a first lodge reaction.
Case ii: Second Order Reaction with Multiple Reactants
Two different reactants (\(\ce{A}\) and \(\ce{B}\)) combine in a single uncomplicated stride:
\[A + B \longrightarrow P \characterization{case2}\]
The reaction rate for this step tin be written as
\[\text{Rate} = - \dfrac{d[A]}{dt}= - \dfrac{d[B]}{dt}= + \dfrac{d[P]}{dt}\]
and the rate of loss of reactant \(\ce{A}\)
\[ \dfrac{d[A]}{dt}= - 1000[A][B]\]
where the reaction order with respect to each reactant is i. This ways that when the concentration of reactant A is doubled, the rate of the reaction will double, and quadrupling the concentration of reactant in a split experiment will quadruple the charge per unit. If we double the concentration of \(\ce{A}\) and quadruple the concentration of \(\ce{B}\) at the same time, then the reaction rate is increased by a factor of 8. This relationship holds truthful for any varying concentrations of \(\ce{A}\) or \(\ce{B}\).
Equally earlier, the charge per unit at which \(A\) decreases can be expressed using the differential rate equation:
\[ \dfrac{d[A]}{dt} = -m[A][B] \nonumber \]
Two situations can be identified.
State of affairs 2a: \([A]_0 \neq [B]_0\)
Situation 2a is the state of affairs that the initial concentration of the 2 reactants are non equal. Allow \(x\) be the concentration of each species reacted at fourth dimension \(t\).
Let \( [A]_0 =a\) and \([B]_0 =b\), then \([A]= a-x\) ;\( [B]= b-ten\). The expression of rate constabulary becomes:
\[-\dfrac{dx}{dt} = -k([A]_o - ten)([B]_o - x)\nonumber \]
which can exist rearranged to:
\[\dfrac{dx}{([A]_o - x)([B]_o - 10)} = kdt\nonumber \]
We integrate between \(t = 0\) (when \(x = 0\)) and \(t\), the time of interest.
\[ \int_0^x \dfrac{dx}{([A]_o - ten)([B]_o - x)} = thousand \int_0^t dt \nonumber \]
To solve this integral, nosotros use the method of partial fractions.
\[ \int_0^x \dfrac{1}{(a - x)(b -x)}dx = \dfrac{1}{b - a}\left(\ln\dfrac{i}{a - x} - \ln\dfrac{one}{b - x}\right)\nonumber \]
Evaluating the integral gives us:
\[ \int_0^x \dfrac{dx}{([A]_o - x)([B]_o - x)} = \dfrac{1}{[B]_o - [A]_o}\left(\ln\dfrac{[A]_o}{[A]_o - x} - \ln\dfrac{[B]_o}{[B]_o - x}\right) \nonumber \]
Applying the rule of logarithm, the equation simplifies to:
\[\int _0^x \dfrac{dx}{([A]_o - ten)([B]_o - x)} = \dfrac{1}{[B]_o - [A]_o} \ln \dfrac{[B][A]_o}{[A][B]_o} \nonumber \]
Nosotros then obtain the integrated rate equation (under the status that [A] and [B] are not equal).
\[ \dfrac{i}{[B]_o - [A]_o}\ln \dfrac{[B][A]_o}{[A][B]_o} = kt \nonumber \]
Upon rearrangement of the integrated rate equation, we obtain:
\[ \ln\dfrac{[B][A]_o}{[A][B]_o} = grand([B]_o - [A]_o)t \nonumber \]
Hence, from the last equation, we tin see that a linear plot of \(\ln\dfrac{[A]_o[B]}{[A][B]_o}\) versus time is characteristic of 2nd-order reactions.
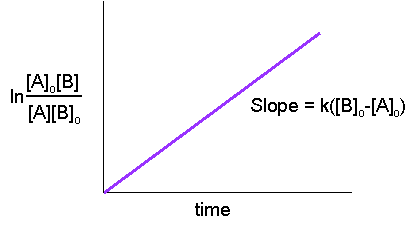
This graph can be used in the same manner as the graph in the section above or written in the other way:
\[\ln\dfrac{[A]}{[B]} = k([A]_o - [B]_o)t+\ln\dfrac{[A]_o}{[B]_o}\nonumber \]
in course \( y = ax + b\) with a slope of \(a= k([B]_0-[A]_0)\) and a y-intercept of \( b = \ln \dfrac{[A]_0}{[B]_0}\)
Situation 2b: \([A]_0 =[B]_0\)
Because \(A + B \rightarrow P\)
Since \(A\) and \(B\) react with a 1 to one stoichiometry, \([A]= [A]_0 -x\) and \([B] = [B]_0 -x\)
at any time \(t\), \([A] = [B]\) and the rate law will exist,
\[\text{rate} = k[A][B] = k[A][A] = chiliad[A]^2.\nonumber \]
Thus, information technology is assumed as the offset case!!!
Instance \(\PageIndex{1}\)
The following chemical equation reaction represents the thermal decomposition of gas \(E\) into \(G\) and \(K\) at 200° C
\[\ce{ 5E(g) -> 4K(chiliad) + Thou(k)} \nonumber \]
This reaction follows a second society charge per unit law with regards to \(\ce{E}\). For this reaction suppose that the rate constant at 200° C is equivalent to \(4.0 \times 10^{-2} M^{-ane}southward^{-one}\) and the initial concentration is \(0.050\; Yard\). Due westhat is the initial charge per unit of decomposition of \(\ce{E}\).
Solution
Start past defining the reaction rate in terms of the loss of reactants
\[ \text{Rate (initial)} = - \dfrac{one}{5} \dfrac{d[E]}{dt}\nonumber \]
and so utilise the charge per unit law to ascertain the charge per unit of loss of \(\ce{E}\)
\[ \dfrac{d[Due east]}{dt} = -k [A]_i^2 \nonumber \]
We already know \(thou\) and \([A]_i\) just we demand to figure out \(x\). To practise this look at the units of \(k\) and 1 sees it is M-1s-1 which means the overall reaction is a second order reaction with \(ten=2\).
\[\brainstorm{marshal*} \text{Initial rate} &= (4.0 \times x^{-2} 1000^{-1}s^{-one})(0.050\,M)^ii \\[4pt] &= 1 \times 10^{-4} \, Ms^{-1}\end{align*} \]
Half-Life
Another characteristic used to decide the order of a reaction from experimental data is the half-life (\(t_{one/2}\)). By definition, the half life of any reaction is the amount of fourth dimension it takes to consume half of the starting cloth. For a second-order reaction, the half-life is inversely related to the initial concentration of the reactant (A). For a second-order reaction each one-half-life is twice equally long as the life span of the one before.
Consider the reaction \(2A \rightarrow P\):
Nosotros tin find an expression for the half-life of a second order reaction past using the previously derived integrated rate equation.
\[\dfrac{1}{[A]_t} - \dfrac{one}{[A]_o} = kt \nonumber\]
Since,
\[[A]_{t_{one/2}} = \dfrac{1}{2}[A]_o \nonumber\]
when \(t = t_{ane/2} \).
Our integrated charge per unit equation becomes:
\[\dfrac{1}{\dfrac{i}{2}[A]_o} - \dfrac{one}{[A]_o} = kt_{1/2} \nonumber\]
After a serial of algebraic steps,
\[\begin{align*} \dfrac{2}{[A]_o} - \dfrac{one}{[A]_o} &= kt_{one/2} \\[4pt] \dfrac{ane}{[A]_o} &= kt_{one/2} \cease{marshal*}\]
We obtain the equation for the one-half-life of a 2nd club reaction:
\[t_{1/two} = \dfrac{one}{k[A]_o} \label{2nd halflife}\]
This inverse relationship suggests that as the initial concentration of reactant is increased, in that location is a college probability of the ii reactant molecules interacting to form product. Consequently, the reactant will be consumed in a shorter amount of fourth dimension, i.eastward. the reaction will have a shorter half-life. This equation also implies that since the half-life is longer when the concentrations are low, species decaying according to second-order kinetics may exist for a longer amount of time if their initial concentrations are small-scale.
Note that for the 2d scenario in which \(A + B \rightarrow P\), the one-half-life of the reaction cannot be determined. As stated earlier, \([A]_o\) cannot be equal to \([B]_o\). Hence, the fourth dimension it takes to swallow one-half of A is not the same as the time it takes to consume one-half of B. Because of this, nosotros cannot ascertain a general equation for the one-half-life of this type of second-social club reaction.
Case \(\PageIndex{ii}\): Half-Life of a Second-Lodge Reaciton
If the only reactant is the initial concentration of \(A\), and it is equivalent to \([A]_0=four.50 \times 10^{-v}\,M\) and the reaction is a second order with a rate abiding \(k=0.89 M^{-i}s^{-1}\). What is the half-life of this reaction?
Solution
This is a direct application of equation \ref{2nd halflife}.
\[\begin{align*} \dfrac{ane}{k[A]_0} &= \dfrac{ane}{(4.l \times 10^{-5} M)(0.89 M^{-1}{s^{-1})}} \\[4pt] &= 2.fifty \times 10^iv \,south \end{align*}\]
Summary
| \(2A \rightarrow P\) | \(A + B \rightarrow P\) | |
|---|---|---|
| Differential Form | \(-\dfrac{d[A]}{dt} = k[A]^2\) | \(-\dfrac{d[A]}{dt} = k[A][B]\) |
| Integral Form | \(\dfrac{1}{[A]_t} = kt + \dfrac{1}{[A]_o}\) | \(\dfrac{1}{[B]_o - [A]_o}\ln\dfrac{[B][A]_o}{[A][B]_o} = kt\) |
| Half Life | \(t_{one/ii} = \dfrac{ane}{chiliad[A]_o}\) | Cannot be easily defined; \(t_{1/2}\) for A and B are dissimilar. |
The graph beneath is the graph that tests if a reaction is second gild. The reaction is second social club if the graph has a straight line, as is in the example below.

Practice Problems
ane. Given the following information, determine the order of the reaction and the value of k, the reaction constant.
| Concentration (One thousand) | Fourth dimension (s) |
|---|---|
| 1.0 | 10 |
| 0.50 | xx |
| 0.33 | 30 |
*Hint: Begin by graphing
ii. Using the following data, decide the one-half life of this reaction, assuming there is a unmarried reactant.
| Concentration (Grand) | Time (s) |
|---|---|
| ii.0 | 0 |
| 1.three | 10 |
| 0.9633 | 20 |
3. Given the information from the previous problem, what is the concentration later 5 minutes?
Solutions
1
- Brand graphs of concentration vs. fourth dimension (zeroth order), natural log of concentration vs. fourth dimension (first order), and one over concentration vs. time (second lodge).
- Make up one's mind which graph results in a straight line. This graph reflects the order of the reaction. For this problem, the directly line should be in the third graph, meaning the reaction is second order.
- The numbers should take are:
| ane/Concentration(G-ane) | Time (s) |
|---|---|
| 1 | 10 |
| 2 | 20 |
| iii | xxx |
The slope can exist found by taking the "ascension" over the "run". This means taking two points, (10,1) and (20,2). The "rise" is the vertical altitude between the points (ii-1=1) and the "run" is the horizontal altitude (twenty-10=10). Therefore the slope is 1/ten=0.1. The value of k, therefore, is 0.one M-2s-i.
ii
- Determine the lodge of the reaction and the reaction constant, k, for the reaction using the tactics described in the previous problem. The order of the reaction is second, and the value of m is 0.0269 One thousand-2s-1.
- Since the reaction club is 2nd, the formula for t1/two = k-ane[A]o -1. This means that the one-half life of the reaction is 0.0259 seconds.
3
- Convert the time (5 minutes) to seconds. This means the time is 300 seconds.
- Apply the integrated rate law to find the final concentration. The final concentration is .1167 One thousand.
References
- Atkins, P. W., & De Paula, J. (2006). Physical Chemistry for the Life Sciences. New York, NY: W. H. Freeman and Company.
- Petrucci, R. H., Harwood, W. S., & Herring, F. G. (2002). Full general Chemical science: Principles and Modern Applications. Upper Saddle River, NJ: Prentice-Hall, Inc.
Contributors and Attributions
- Lannah Lua, Ciara Murphy, Victoria Blanchard
Second Order Kinetics Half Life,
Source: https://chem.libretexts.org/Bookshelves/Physical_and_Theoretical_Chemistry_Textbook_Maps/Supplemental_Modules_(Physical_and_Theoretical_Chemistry)/Kinetics/02%3A_Reaction_Rates/2.08%3A_Second-Order_Reactions#:~:text=Since%20the%20reaction%20order%20is,the%20reaction%20is%200.0259%20seconds.
Posted by: joneslessed.blogspot.com


0 Response to "Second Order Kinetics Half Life"
Post a Comment